If G is abelian, then G is of symmetric type, that is, there exists a group K such that G is isomorphic to the direct product KxK. Conversely, any finite abelian group of symmetric type KxK is an index group.

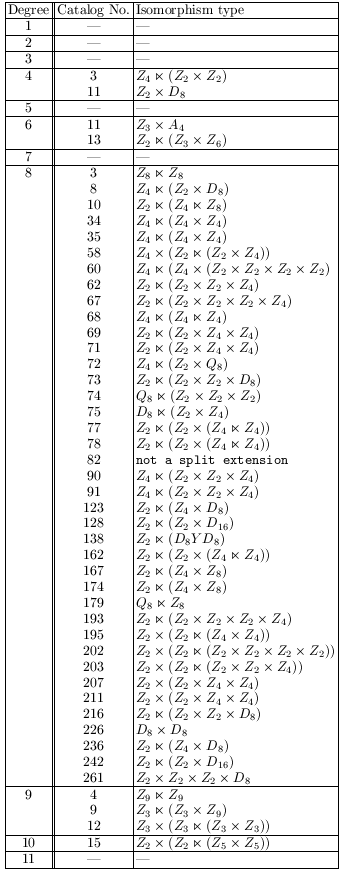
Nonabelian index groups G can only exist if the degree n is not a prime. It can be shown that G is a solvable group. The following table gives a complete list of the nonabelian index groups of order 121 or less. The first column gives the degree n of the bases, thus G is of order n^2. The second column gives the number in the catalogue of small groups used in GAP3, GAP4, and MAGMA.